Correspondents raise instances of damage to baluns with me from time to time, and there is a steady stream of reports online.
One of the very common reports is of something unexpected happening while adjusting an ATU, after perhaps 30s of power applied, VSWR suddenly becomes unstable, changing for some unknown reason, and attempts to find optimal settings of the ATU fails.
A likely cause of this is non-linear behavior of the ferrite core in a balun in the system.
Let’s talk about that.
A theoretical model of temperature rise
A simple model that gives useful insight is to consider the case of a toroid core in still air, being heated by constant applied RF power giving rise to core loss.
Core temperature rises quickly initially, then more slowly as the core heats up and loses more and more heat to the surrounding air.
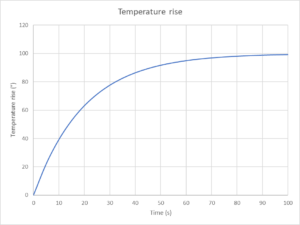
We can write and expression for core temperature T: \(T=T_{max}\left(1-\mathrm{e}^{-\frac{t}{\tau}}\right)\) where τ is the thermal time constant and Tmax is the final temperature if things continued without disruption.
Above is an example where τ=20s and temperature rise is 100°.
Note that at the beginning (t=0), the slope of the line is pretty constant, but as temperature increase, slope decreases until eventually it is almost zero.
The slope can be calculated by differentiating the expression above: \(\frac{dT}{dt}=\frac{T_{max}\mathrm{e}^{-\frac{x}{\tau}}}{\tau}\).
At t=0, \(\frac{dT}{dt}=\frac{T_{max}}{\tau}\), \(T_{max}=\tau \frac{dT}{dt}\).
From the graph, by eye we can estimate the slope near t=0 to be about 5°/s and τ=20 (the time to reach 63% of Tmax) and calculate that Tmax is 5*20=100°.
Applying the model to a practical example
Let’s now look at a practical device using ferrite toroid using a FT240-43 core initially at 30°.
Ferrite materials are subject to Curie effect, above a certain temperature (known as the Curie temperature) they rapidly lose their magnetic properties (temporarily or permanently).
For #43 material, that is specified at 130° or higher.
We can also measure the thermal time constant τ for the FT240 core, and find τ=2000.
We apply RF power and find that after 30s, the indicated VSWR changes without external change, the core has reached its Curie temperature and is losing its magnetic properties.
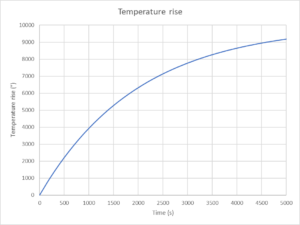
Because the time observed, 30s, is very small compared to τ=2000, we can reasonably estimate the slope of the response near t=0 as \(\frac{T_{rise}}{t}=\frac{100}{20}=5 \text{°/s}\) and go on to calculate \(T_{max}=\tau \frac{dT}{dt}=2000 \cdot 5=10000 \text{°}\).
Theoretically, the complete temperature curve would look like this.
The core will never reach Tmax, temperature increase is terminated when the Curie temperature is reached and the core will be at 130° or more… likely to be sufficient to cause damage to wire insulation and enclosure.
There is something seriously wrong!
Conclusions
The observation of this behavior is a warning that something is not right, and system components should be carefully inspected for faults and possible consequent damage (eg overheating of a ferrite core as a result of some other fault).