This article explores the concept of the Effective Aperture, Capture Area or Effective Area of an antenna.
If some power Pt is radiated from source equally in all directions, then at some distance r from the source, the power is distributed evenly in the sphere which has a surface area of 4πr2, and the Power Flux Density (PFD) therefore is Pt/(4πr2) W/m2.
It could be taken that some received power Pr must be 'captured' from an effective area or aperture Ae of Pr/(Pt/(4πr2)) m2 ...(1).
Harald Friis showed that the ratio of received power to transmitted power
between two unity gain antennas separated by distance r in free space is given by
Pr/Pt=(λ/(4πr))2
m2...(2).
By substituting (2) into (1), we find that Ae
for a unity gain antenna is λ2/(4π).
A receive antenna with gain Gr has
Ae=Grλ2/(4π).
So, we can speak of the effective aperture Ae, and we can calculate the receive power knowing the Ae and PFD.
(IEEE1993) defines effective area (of an antenna) (in a given direction).
In a given direction, the ratio of the available
.
power at the terminals of a
receiving antenna to the power flux density of a plane wave incident on the
antenna from that direction, the wave being polarization matched to the antenna
It is tempting to visualise Ae as some plane area that one could run a ruler over and measure, but it doesn't work that way for most antennas.
Taking the common half wave dipole which has a gain of 1.64 (2.15dB), Ae=1.64λ2/(4π)=0.13λ2. For example, Ae for a lossless 7MHz half wave dipole is about 240m2, whereas the projected area of the dipole conductors is more like 0.04m2. Clearly you cannot run a tape measure over the dipole to obtain its effective aperture.
There are some types of antennas where the effective aperture is closely related to projected area. A parabolic dish is one, for an ideal lossless parabolic antenna the effective aperture is equal to the projected area... but sadly, real antennas are less than ideal, and the effective aperture is more like 50% to 60% of the projected area for a good parabolic antenna.
Though it might be tempting to evaluate the effective aperture of a λ/4 a side loop as 0.062λ2, the effective aperture of a lossles loop of that size is almost three times as much at more like 0.17λ2.
There is nothing wrong with the concept of effective aperture, or capture area. The failing is in thinking that you can measure up the conductors of any antenna and simply calculate the effective aperture from the projected area. What you can do is calculate the effective aperture from known gain, Ae=Grλ2/(4π).
 |
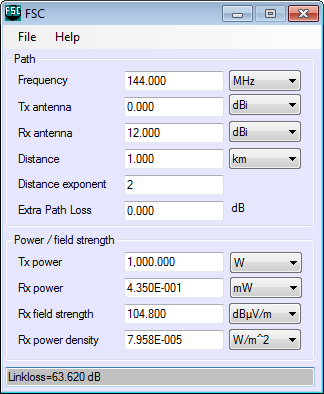
Above is a screen grab from FSC. It shows calculation of a free space path on 144MHz, with a 1kW transmitter and unity gain antenna (0dBi) and a receiver at 1km with a 12dB (15.85) gain antenna.
The PFD at 1km is Pt/(4πr2) =1000/(4π10002)=7.958E-5 W/m2.
The effective aperture is Grλ2/(4π)=15.85*2.0832/(4π)=5.47m2.
Receive power is PFD*Ae=7.958E-5*5.47=0.435mW.
Loss reduces antenna system gain, and so it reduces antenna system effective aperture.
For example, if I have an 80m half wave dipole made from 1mm diameter copper conductor, and I replace it with one of the same length of 2mm diameter steel conductor, the latter will have more loss and lower effective aperture, yet exactly the same physical size. Another example of why effective aperture of real antennas cannot simply be determined from their physical dimensions.
The following calculator will find Ae given frequency and gain.
Gain must be specified in dB relative to a lossless antenna that radiates
equally in all directions, or dBi. (IEEE 1993) states [t]he effective area of
an antenna in a given direction is equal to the square of the operating
wavelength times its gain in that direction divided by 4pi
.
The calculator does not do a lot of error checking, if you enter nonsense, it will produce nonsense. NaN means not a number, check the input values.
A posting recently in QRZ forums, explained to the gullible audience that the area concept could be readily translated into antenna gain. The poster stated that:
gain of a loop is directly related to the enclosed area of the loop.
You might have read that to mean that gain is proportional to area. Lets look at that, the gain of a very small lossless square loop (side < λ/10) is 1.5 and almost independent of its size, and the effective aperture is therefore 1.5λ2/(4π)=0.12λ2, again almost independent of size.
Busted!
He went on:
However, as a first order of COMPARATIVE approximation, the "area" of a dipole can be considered a square with the edge equal to the length of the dipole. So a ten meter 1/2 wave dipole would have an "aperture area" of about 5 meters by five meters or 25 square meters. Again, this is only for comparative purposes, not as an absolute factor.
Well, the (maximum) gain of a lossless half wave dipole is 1.64, Ae=1.64λ2/(4π)=0.13λ2, so at 10m, a half wave dipole (oriented for maximum signal) has an effective aperture of 13m2, so much for the poster's first approximation which has +92% error. (The effective aperture of a practical 10m half wave dipole will be slightly less, perhaps 1%, due to conductor loss.) As discussed above, you cannot use a ruler to measure the sides of the effective aperture of a half wave dipole, it is a virtual area.
Busted!
In an earlier but related post he treated us to:
The more copper (or aluminum) you have up in the air, the better your station will work. This is a corollary of the NFL parameter (No Free Lunch).
Fat dipoles work better than skinny dipoles. I remember hearing this ages ago, but hadn't paid much attention to it until I started working at HIPAS Observatory and HAARP; our findings were also confirmed by the fine work of W4RNL on his second book of Quads. The increase in bandwidth is not the only reason to use fatter antenna elements. It can be consistently demonstrated by measuirement and modelinig, that a fat dipole can have over 1dB of gain over a thin wire dipole.
Though it is padded with irrelevant details to make a narrative that is swallowed hook line and sinker by the gullible, the claim that a fat dipole can have over 1dB better gain than a thin wire dipole is very misleading. If a half wave dipole for 40m say is constructed of copper conductor that is adequate to withstand high winds, doubling the diameter (ie four times the mass of copper) will make much less than 1dB increase in gain. Later in the thread, he seems to allow that the NEC model on which he based his 1dB claim may be a flawed model.
Busted!
And getting carried away with the mistaken concept of capture area:
Figuring out the OAOS of a long single yagi is a little trickier, but if you were to take the total aperture area of each element, it will have close to the same gain as a loop with the same enclosed area.OAOS is the poster's new jargon,
Occupied Area of Sky. This would imply that capture area and hence gain in a Yagi is simply proportional to the number of elements. That is not the case, in the case of long Yagis, gain is a function of boom length provided they have sufficient elements. Adding more than sufficient elements does not signficantly increase gain or capture area, much less increase it proportional to the number of elements.
Busted!
Stay tunedIndeed, for more ham insights into antennas, a perspective you are not likely to find in reputable text books.
Another poster on QRZ forums...
An example of antenna area is this, A 80 meter dipole has 7000 times the area to be induced with a signal that a 2 meter dipole has yet both work as they should for each band
The effective aperture of two antennas of the same gain is proportional to the wavelength squared, so in this case (80/2)^2=1600, not 7000 as stated.
Busted!
The simple reality is that bigger isn't necessarily better, and appealing explanations are correct simply because they are appealing.
The quiet acceptance of this nonsense by hams is a sign of the dumbing down of ham radio. Indeed QRZ's Chief Editor posted his thought that it was a post worthy of QRZ.com forums.
| Version | Date | Description |
| 1.01 | 05/06/2011 | Initial. |
| 1.02 | 09/06/11 | Added inline calculator. |
| 1.03 | ||
| 1.04 | ||
| 1.05 |